
Getting old gracefully, and risk of dying before getting there: a new guide to theory on extrinsic mortality and senescence
Extrinsic mortality and senescence: a guide for the perplexed
Abstract
Recommendation: posted 06 March 2023, validated 06 March 2023
English, S. and Nakagawa, S. (2023) Getting old gracefully, and risk of dying before getting there: a new guide to theory on extrinsic mortality and senescence. Peer Community in Evolutionary Biology, 100626. https://doi.org/10.24072/pci.evolbiol.100626
Recommendation
Why is there such variation across species and populations in the rate at which individuals show wear and tear as they get older? Several compelling theoretical explanations have been developed on the conditions under which selection allows for or prevents senescence; a notable one being that proposed by George C Williams in 1957 based on the idea of antagonistic pleiotropy (Williams, 1957). One of the testable predictions of this theory is that, in populations where adults experience higher mortality, senescence is expected to be faster. This is one of the most influential predictions of the paper, being intuitive (when individuals are less likely to survive to later age classes, we expect weakened selection on traits that would avoid senescence in these classes), and fitting with ‘live fast, die young’ life history framing. As such, it has been widely incorporated into how we think about the evolution of senescence and has received considerable support in comparative studies across species and populations.
However, it would be misleading to sit back at this point and think we have ‘solved’ the problem of understanding variation in senescence, and how this is linked with mortality. It turns out that the Williams 1957 paper is hotly contested by theoreticians: for the past 30 years – with increasing focus in the last 4 years – a growing body of models and opinion pieces have proposed flaws in the paper itself and in how it has been interpreted (Abrams, 1993; André and Rousset, 2020; Day and Abrams, 2020; Moorad et al., 2019). Central to several of these critiques is that explicit consideration of density dependence (not considered in Williams’ original paper) changes the conditions under which his predictions hold. A new preprint by de Vries, Gallipaud and Kokko brings further clarity to such critiques of the original paper (Vries et al., 2023).
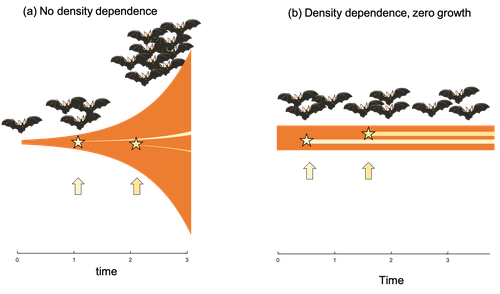
Beyond just continuing the tradition of critiquing Williams’ prediction, however, de Vries et al. provide a clear guide that is accessible to non-theoreticians about the issues with William’s prediction, and the way in which density dependence and how it operates can change when we expect senescence to occur. Rather than reiterate their points here, we suggest a close reading of the paper itself, along with an excellent overview of the paper in a recent blog by Daniel Nettle (Nettle, 2022). In brief, the paper starts by synthesizing earlier theoretical and empirical studies on the topic and presenting a very simple model to highlight how – in the absence of density dependence – Williams’ prediction does not hold because of the unregulated population growth, which is necessarily higher when there is low mortality. As a result, for a lineage with low mortality, any fitness advantage of placing offspring into the lineage later (i.e. selection for reduced senescence) is exactly cancelled out by the fact that earlier-produced offspring have higher fitness returns.
They then present a more complex framework, which incorporates realistic mortality distributions, trade-offs between survival and reproduction, and use a series of 10 scenarios of density dependence (and whether this acts on survival or fecundity, and also whether it depends on a threshold or stochastic, or exerts continuing pressure on the trait) to explore selection on fitness-associated traits with age depending on extrinsic mortality. This then generates a range of results for when the Williams prediction holds, when there is no link between mortality and senescence, and when there is an ‘anti-Williams’ result – i.e., where senescence is slower when there is a high mortality. As has been shown in earlier studies, density dependence and how it operates matters, and Williams’ prediction holds most when density dependence affects juvenile age classes (in their model, when adults are less likely to produce them – i.e. there is density dependence on fecundity; or when there is less recruitment into the adult population due to, for example, competition among juveniles).
So, perhaps we are now at a point where we can lay to rest the debate on the issues specifically with Williams’ original paper, and instead consider more broadly the key factors to measure when predicting patterns of senescence, and what is tangible for empiricists to quantify in their studies. Here, de Vries et al. provide some helpful insights both into the limitations of their approach and what modelling should be done moving forward, and into how we can link existing studies (for example comparing senescence among populations with varying predation pressure) to the theoretical predictions. At the heart of the latter is understanding the mechanism of density-dependent regulation – does it affect survival or fecundity, which age classes are most sensitive, and how do key traits depend on density? – and this is often difficult to measure in field studies.
And from all this we can learn that even very intuitive and extensively discussed concepts in biology do not always have as firm theoretical underpinnings as assumed, and – as should not be surprising – biology is complex and rather than one clear pattern being predicted, this can depend on a multitude of factors.
REFERENCES
Abrams PA (1993) Does increased mortality favor the evolution of more rapid senescence? Evolution, 47, 877–887. https://doi.org/10.1111/j.1558-5646.1993.tb01241.x
André J-B, Rousset F (2020) Does extrinsic mortality accelerate the pace of life? A bare-bones approach. Evolution and Human Behavior, 41, 486–492. https://doi.org/10.1016/j.evolhumbehav.2020.03.002
Day T, Abrams PA (2020) Density Dependence, Senescence, and Williams’ Hypothesis. Trends in Ecology & Evolution, 35, 300–302. https://doi.org/10.1016/j.tree.2019.11.005
Moorad J, Promislow D, Silvertown J (2019) Evolutionary Ecology of Senescence and a Reassessment of Williams’ ‘Extrinsic Mortality’ Hypothesis. Trends in Ecology & Evolution, 34, 519–530. https://doi.org/10.1016/j.tree.2019.02.006
Nettle AD (2022) Live fast and die young (maybe). https://www.danielnettle.org.uk/2022/02/18/live-fast-and-die-young-maybe/ (accessed 2.27.23).
de Vries C, Galipaud M, Kokko H (2023) Extrinsic mortality and senescence: a guide for the perplexed. bioRxiv, 2022.01.27.478060, ver. 5 peer-reviewed and recommended by Peer Community in Evolutionary Biology. https://doi.org/10.1101/2022.01.27.478060
Williams GC (1957) Pleiotropy, natural selection, and the evolution of senescence. Evolution, 11, 398–411. https://doi.org/10.1111/j.1558-5646.1957.tb02911.x
The recommender in charge of the evaluation of the article and the reviewers declared that they have no conflict of interest (as defined in the code of conduct of PCI) with the authors or with the content of the article. The authors declared that they comply with the PCI rule of having no financial conflicts of interest in relation to the content of the article.
This research was supported by Swiss National Science Foundation grant number 310030B_182836 (awarded to Hanna Kokko). CdV was also supported by an Academy of Finland grant (no. 340130, awarded to Jussi Lehtonen).
Evaluation round #1
DOI or URL of the preprint: https://doi.org/10.1101/2022.01.27.478060
Version of the preprint: 3
Author's Reply, 20 Jan 2023
Dear recommender/PCI,
Please consider the resubmission of our manuscript, titled “Extrinsic mortality and senescence: a guide for the perplexed”, for publication in PCI Evolutionary Biology. We are very grateful for the feedback and suggestions. We have tried to implement (almost) all suggestions, and we believe it has significantly improved the manuscript. We provide detailed responses to the reviewers in the attached document, and we have also attached a document with tracked changes.
Thank you for your consideration,
The authors
Decision by Sinead English, posted 07 Oct 2022
Dear Dr de Vries and co-authors
I have read with great interest your manuscript on the theory behind Williams' prediction about the association between reduced extrinsic mortality and lower senescence, and the layered approach to presenting scenarios in which such prediction holds and when it does not. I can see this being an important contribution to the literature on this topic which will bring clarity to the arguments for and against Williams' predictions; and will also make this accessible to a broader readership including those who may be hesitant to engage with more dense theoretical papers on the subject.
The manuscript has been appraised by two reviewers who are both enthusiastic about the premise and aims of the manuscript. That said, they both also provide a series of detailed suggestions to further improve clarity and - having read through these - I entirely agree, and do not have further revisions to add myself. In particular, I agree with Reviewer 1 (Walasek) that it would strengthen the reach of this paper to give more background explanation of previous models (rather than assuming the reader is familiar with these), and to clarify terms such as 'selection gradient' which will make the already quite accessible theory in this manuscript even more digestible to non-theoreticians. Reviewer 2 also makes a number of helpful suggestions to improve clarity both of the text and the figures.
I look forward to reading the revised manuscript if the authors are in agreement with these suggestions.
Yours sincerely,
Sinead English
Reviewed by anonymous reviewer 1, 29 Sep 2022
Dear authors,
Please find my review of the manuscript, “Extrinsic mortality and senescence: a guide for the perplexed”.
General comments:
The authors firstly present examples focusing on the occurrence of a null result (where extrinsic mortality has no effect) and the importance of the presence/absence of density-dependence. They then investigate 10 differing scenarios that incorporate density-dependent effects on either age-independent survival, survival at old ages or recruitment. This is in addition to also considering three different forms of density-dependence (deterministic, stochastically or continuous). Ultimately, the authors show that under these different conditions, three scenarios can occur, namely, the null model, the Williams prediction (where increasing extrinsic mortality leads to the evolution of faster senescence) and the anti-Williams model (where increased extrinsic mortality leads to the evolution of slower senescence).
Overall, I believe this to be an important and well-written paper and really only have comments regarding the need for additional clarification and increasing the interpretability of some of the figures, mainly Figure 5 (where the results from the ten different scenarios are shown).
Specific comments:
Keywords: is it worth also adding density-dependence and slow-fast continuum keywords here?
L22: Here and elsewhere: keep with either numerical numbers or written numbers e.g., “ten” – so it matches L86 or for instance on L176 - “one”.
L24: Possibly worth changing, “that vary along the fast-slow continuum”.
In addition, could this be simplified to “favour life histories that vary along the fast-slow continuum”, as it is implied that they must either be slow or fast?
L25-28: “could suggest” sounds odd here. I would consider trying to rewrite this sentence for clarity and possibly end with more of a summarising sentence to this abstract.
L58: Could this be reworded to something simpler: i.e., “prediction made by Williams holds and also scenarios when it doesn’t hold? – or something similar”.
L77: Slight reword to: “by instead focusing explicitly”.
L85: Does the use of Gompertz-Makeham survival curves need a very brief explanation? I guess this is discussed on L375 but might be useful here too when it’s first introduced.
L117: Comma after “we are” and after” in this first exercise”.
L124: Is it worth making clear that the bat and mouse can be both fast or slow. Currently the way it is worded could sound like both species have competing life histories (at least to me). For instance - “Both the bat and the mouse are able to be either one of two competing life histories that differ…”.
L128-129: What does “The sign of selection is therefore clear” mean. Possibly reword.
L158-159: This is a really important point to make.
L173: Here you might want to reiterate that fast senescers only breed once which is why you’re focusing on slow senescers and the ability to breed twice.
L218-221: Not sure I follow this sentence, potentially worth rewriting for clarity.
L230: Unless I’m mistaken, should this be 0.6/3.51? Should the notation be SM or SB?.
L371-372: Could this be rephrased to: “we contrasted the success of a fast life history that senesces, with a slow life history that does not experience senescence”.
L378: (Not a comment, just a thought) I wonder what would happen if fecundity was allowed to change either instead of survival or at the same time, i.e., followed an age-specific reproductive schedule with peak reproduction in midlife followed by a senescent decline?
L379: Worth reiterating that these are 3A-D in Tables 2/3.
L381: If these are indeed steps - would it be more appealing to place them in a numbered order and then also link them to the life cycle on Figure 4. So the readers know which section describes what part of the standard procedure.
L430: Here and elsewhere: density-dependence/dependent with or without a hyphen. Keep consistent.
448-459: This is a very important paragraph. It would be good if this section could directly refer to Figure 5. i.e., when discussing 1A-1C, you could say exactly why the lines represent the null result with reference to values of F0 and F1? This could make it a bit easier to follow. Same with the Williams and anti-Williams mentions.
L481-495: This is also an important paragraph that helps readers understand why a Williams pattern is seen, perhaps you could also add some further explanation about 2A-C and why anti-Williams might be seen?
Figure 1-2: Could there be an indication on the graph that the width of line indicates payoff.
I would keep with the notation of time in both figures (Figure 1 preferably), just for consistency.
Figure 3: What does a and d represent here (I realise it's mentioned earlier, but worth making it obvious in the legend)
Figure 4: “take the same amount of time” rather than “takes equally much time”.
Reword “to allow mortality rates the appropriately long time to apply before the next year”.
Figure 5 is an important graph. I think it could do with some adjustments as currently it’s a tad confusing (though could be a personal preference). For instance:
- In the legend, reiterate that slow = F0 and fast = F1.
- The lines could do with some better explanation and could be clearer. Something like “threshold fecundities for the slow type to win when F1 fecundity differs” or something similar.
- Also, you could probably remove some of the axis labels as they are repeated row/column wise, which may reduce clutter?
- 3C also needs to include 1 as an axis limit as currently it looks to be a bigger increase than the others but really could be a scaling issue.
- Also, perhaps it could be easier to move the legend to below the graph, so it doesn’t look like its related to the “competition for territories” column, unless you place the title directly over 3D.
- Also, unless I missed this, what was the justification for these values of F1 fecundity and for the a/d parameters of the Gompertz-Makeham (i.e., had 5 possible values and d had 2)? Just some additional clarification could be useful (in text and possibly legend would be ideal).










