
Two parasites, virulence and immunosuppression: how does the whole thing evolve?
Co-evolution of virulence and immunosuppression in multiple infections
Abstract
Recommendation: posted 14 December 2017, validated 18 December 2017
Magalhaes, S. (2017) Two parasites, virulence and immunosuppression: how does the whole thing evolve?. Peer Community in Evolutionary Biology, 100043. https://doi.org/10.24072/pci.evolbiol.100043
Recommendation
How parasite virulence evolves is arguably the most important question in both the applied and fundamental study of host-parasite interactions. Typically, this research area has been progressing through the formalization of the problem via mathematical modelling. This is because the question is a complex one, as virulence is both affected and affects several aspects of the host-parasite interaction. Moreover, the evolution of virulence is a problem in which ecology (epidemiology) and evolution (changes in trait values through time) are tightly intertwined, generating what is now known as eco-evolutionary dynamics. Therefore, intuition is not sufficient to address how virulence may evolve.
In their classical model, Anderson and May [1] predict that the optimal virulence level results from a trade-off between increasing parasite load within hosts and promoting transmission between hosts. Although very useful and foundational, this model incurs into several simplifying assumptions. One of the most obvious is that it considers that hosts are infected by a single parasite strain/species. Some subsequent models have thus accounted for multiple infections, generally predicting that this will select for higher virulence, because it increases the strength of selection in the within-host compartment.
Usually, when attacked, hosts deploy defences to combat their parasites. In many systems, however, parasites can suppress the immune response of their hosts. This leads to prolonged infection, which is beneficial for the parasite. However, immunosuppressed hosts are also more prone to infection. Thus, multiple infections are more likely in a population of immunosuppressed hosts, leading to higher virulence, hence a shorter infection period. Thus, the consequences of immunosuppression for the evolution of virulence in a system allowing for multiple infections are not straightforward.
Kamiya et al.[2] embrace this challenge. They create an epidemiological model in which the probability of co-infection trades off with the rate of recovery from infection, via immunosuppression. They then use adaptive dynamics to study how either immunosuppression or virulence evolve in response to one another, to then establish what happens when they both coevolve. They find that when virulence only evolves, its evolutionary equilibrium increases as immunosuppression levels increase. In the reverse case, that is, when virulence is set to a fixed value, the evolutionarily stable immunosuppression varies non-linearly with virulence, with first a decrease, but then an increase at high levels of virulence. The initial decrease of immunosuppression may be due to (a) a decrease in infection duration and/or (b) a decrease in the proportion of double infections, caused by increased levels of virulence. However, as virulence increases, the probability of double infections decreases even in non-immunosuppressed hosts, hence increased immunosuppression is selected for.
The combination of both Evolutionary Stable Strategies (ESSs) yields intermediate levels of virulence and immunosuppression. The authors then address how this co-ESS varies with host mortality and with the shape of the trade-off between the probability of co-infection and the rate of recovery. They find that immunosuppression always decreases with increased host mortality, as it becomes not profitable to invest on this trait. In contrast, virulence peaks at intermediate values of host mortality, unlike the monotonical decrease that is found in absence of immunosuppression. Also, this relationship is predicted to vary with the shape of the trade-off underlying the costs and benefits of immunosuppression.
In sum, Kamiya et al. [2] provide a comprehensive analysis of an important problem in the evolution of host-parasite interactions. The model provides clear predictions, and thus can now be tested using the many systems in which immunosuppression has been detected, provided that the traits that compose the model can be measured.
References
[1] Anderson RM and May RM. 1982. Coevolution of hosts and parasites. Parasitology, 1982. 85: 411–426. doi: 10.1017/S0031182000055360
[2] Kamiya T, Mideo N and Alizon S. 2017. Coevolution of virulence and immunosuppression in multiple infections. bioRxiv, ver. 7 peer-reviewed by PCI Evol Biol, 149211. doi: 10.1101/139147
The recommender in charge of the evaluation of the article and the reviewers declared that they have no conflict of interest (as defined in the code of conduct of PCI) with the authors or with the content of the article. The authors declared that they comply with the PCI rule of having no financial conflicts of interest in relation to the content of the article.
Evaluation round #2
DOI or URL of the preprint: 10.1101/149211
Version of the preprint: 3
Author's Reply, 08 Dec 2017
Please see the attached PDF document.
Decision by Sara Magalhaes, posted 15 Nov 2017
Dear Authors
I think that this new version of the manuscript has greatly improved in clarity and, because the science was already very good, we are at the verge on publishing a recommendation for this article. I do however have some minor points that you could address in this new version, if you agree. Here it goes:
- Title: wouldn’t it be ‘in’ rather than ‘through’?
- An article on the classical host-parasite interaction of rabbits and myxoma virus came out (Kerr PNAS 29 aug 2017) describing a novel strain that immunosuppresses the host, maybe worth mentioning?
- Abstract line 7: I would put “host-parasite interactions”.
- Abstract line 17: “the shape of the trade-off determining the cost and benefit of immunosuppression” is not clear. What is being traded off with what? Maybe rephrase here.
- Line 36: help maintain -> helps maintaining.
- You state that immunosuppression translates into a longer infection period (eg line 43). Can’t we imagine that, instead, it leads to a higher parasite load for the same time period?
- Line 68: again, trade-off between what and what?
- Line 83: replace “the single species model” by “it”.
- Line 84: replace “than” with “which is not the case for”.
- Line 101: remove extra space before “doubly”.
- Legend of Table 1: put a comma after “evolve”.
- Line 168: replace “evolutionarily” by “evolutionary”.
- Line 188: replace “in which” by “therefore”.
- Line 201: again, trade-off between which variables?
- Line 242: I also found Koella and Boete 2003 Am Nat, I let you decide whether it is sufficiently relevant within this context.
- I still find figure 4 a bit cryptic but have no obvious suggestion for improvement…
Evaluation round #1
DOI or URL of the preprint: 10.1101/149211
Version of the preprint: 2
Author's Reply, 25 Oct 2017
Decision by Sara Magalhaes, posted 14 Jul 2017
The article presents a model analyzing the co-evolution of immunosuppression and virulence under multiple infections. This represents a very important contribution for the existing models on both the evolution of virulence under multiple infections and the evolution of virulence in immunosuppressing parasites (with the latter being clearly less explored than the former). The model is very well-designed and provides valuable insight into this important question. However, the reviewers and myself found that the presentation of several results was hard to follow. Also (I do not discard that this may be related to the previous sentence) some parameter choices seem rather arbitrary, or at least unjustified. I understand that the issue at stake is a complex one, with several factors operating simultaneously. However, it is important for the reader to take some message home… This is compromised at several instances, figure 3 being a paradigmatic example of this (cf. comments by rev1). On a more positive note, I think the discussion does a good job in summarizing the main findings. Overall, I’m convinced of the high quality of this manuscript, but would urge the authors to consider the reviewers comments to improve the clarity of their message. Below I also present my own comments.
Main comment:
As mentioned in lines 187-190 and 211-216, and recapitulated in the Discussion (lines 282-284) double infections are protected from further infections. In my opinion, this may be the crucial factor of your model. That is, would you allow for triple infections (or more than that), wouldn’t high ESI at low virulence pattern vanish? Actually, the situation found here is much akin to models of niche construction (immunosuppression can be considered as a case of niche construction), in which the evolution of this trait is favored if niche constructers also evolve means to monopolize the resource (e.g., Krakaeur et al. 2009 Am Nat). Maybe establishing this parallel would be useful?
Minor comments:
Line 15: The existence of different host types comes as a surprise… which types of hosts are there?
Line 27: any reason not to cite Van Baalen and Sabelis 1995 here?
Lines 31-34: I find the transition quite abrupt here. In the abstract you mention that most models do not consider the coevolution of traits in parasites. Maybe you could explore this a bit more here before turning to the particular traits you will tackle?
Lines 35-43: I know this is a bit biased, but if you would cite some examples from the plant-parasite literature, you would also attract more attention from that community, which unfortunately often neglects host-parasite theory. Eg Sarmento et al. Ecol Lett 2011, Burgyan and Havelda 2011 Trends Plant Sci. This can also be done in the Discussion section.
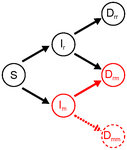
Lines 79-80: equations: why is the recovery rate of doubly infected to single infected the double of that from singly infected to susceptible (2 γ Drr vs γ Ir)?
Line 159: “convergently stable”, instead of “convergent stable” (cf also relevant comments of rev1 on this part).
Line 166: I would remove “game theoretically”.
Line 177: remove “assumption”.
Figure 1: Is there a specific reason for not exploiting the whole range of virulence? Especially, the slight increase observed for higher virulence values makes us wonder what will happen for even higher values. Figs 1d,e: I understand space is limited but maybe stating ‘relative frequency of (co)infected hosts’ in the Y axis would be much more intuitive… Also please consider a comment by rev1 on the trade-off values adopted here. Note also that the δ values stated here differ from those in table 1.
Lines 187-190: this section needs some streamlining, as the information on low virulence being correlated with more double infections is provided twice.
Reviewed by anonymous reviewer 2, 05 Jul 2017
I found the paper interesting and well-written, and I enjoyed reading it.
One concern I have though is that Figure 3 and the results it presents are quite hard to parse for the readers. The color levels are not explained in the legend or in the Figure: what is being plotted? The default set of parameters (used in all results and figures before) appears quite atypical: quite decelerating for one trade-off and quite decelerating for the other. What motivates this particular choice? Why not take, if any, a "simple" reference point such as linear/linear? It is very important that the readers understand to what extent all that is said in the first part of the results (Figs 1& 2) is robust to the trade-off curves, and not specific to the values 0.25 and 0.05 that were chosen. Figure 3 should be made more readable but otherwise makes a good job at showing how the trade-off parameters affect evolutionary dynamics. However, it only reports evolutionary stability (ESS / convergence stability), not the location of the (co)ESS. I would expect the location of the co-ESS, which is obviously the main focus of the article, to receive the same treatment and be explored over a range of tradeoff shapes. As it stands, the first part details results on coESS location for a specific set of trade-off functions, and then we get a generalization to other tradeoff functions, but only for evolutionary stability. It seems that the section about trade-off shape and evolutionary stability, restricted in fact to Figure 3 and to less than 20 lines at the end of the Results (l. 220-236) are quite disconnected. And the relative length of the latter part, especially considering the more complex Figure 3, make it underrepresented. This is also appearant in the Discussion, where line 253 we just have one sentence "In addition, immunosuppression evolution is influenced considerably by the precise shape of the trade- offs determining the cost and benefit of immunosuppression" to sum up these findings. I think the authors should adopt the same approach (i.e. consider a range of trade-off shpes) for all results (both the location of the (co)ESS and the evolutionary stability), and also restore some balance between the attention given to evolutionary stability versus ESS location. Considering there are only three figures, there is ample space for one or two additional figures, if needed (e.g. if a large part of the results are worked out for a specific shape of tradeoff functions, then it can be worth plotting these specific fucntions as the firt figure; currently we only have a general presentation of all possible tradeoff shapes and it is only in the Supp. Material).
I also have some more technical questions/remarks on the model and its presentation:
-a- On multiple infection and omission of Dmm: the authors motivate this omission from the rarity of the mutant. This is a common assumption when individuals mix randomly and thus the probability of encountering another mutant is vanishnigly small. However, in this model, what is a multiple infection exactly? Can a secondary infection originates from the host itself? I mean, if multiple infections are infections at different parts of the body or different tissues, could not a virus reinfect its own host? I would think of left lung infection to right lung infection in humans, or infection of a different master twig in a tree-crown. This would actually be the most likely route to multiple infection considering the physical proximity. When this is the case, the fact that a mutant is very rare does not compromise the rate of multiple infection so much, and so Dmm sould not be neglected. I presume the authors have in mind that a second mutant should necessarily come from a different host, which motivates the assumption, but why would it be necesssarily so? Perhaps the authors can elaborate on motivating their assumption, in relation to the biological mechanisms considered. I think more generally the definition of a multiple infection deserves some attention/explanation. Indeed, the examples provided are mostly for very different infectious agents (e.g. the helminth that favors microparasites through immunosuppression, l. 48). In contrast, if I understand the model considers very similar (or perfectly similar) strains of the same pathogen: Drr or Dmm denote double infection from the same variant, end even r and m are marginally different variants. In this context, how could Dmm not be the same as Dm? My understanding is that this implies that we can still discriminate the two m populations in Dmm, which would in turn mean (if they are genetically identical) that they form two physically distinct subpopulations in one same host (and see the previous paragraph on what this would imply for the omission of Dmm). Otherwise, it would mean that adding a very small amount of propagule from the same genotype would considerably alter the within-host population dynamics, which is not very intuitive to me.
I am sure the authors can clarify all these points and discuss them, and the key is to specify more precisely what is the within-host population dynamics. This is important because several modelling assumptions (and some results it seems) hinge on that: for instance, the fact that \alpharr is the same as \alphar, or that \alpharm is the average of the \alpha, suggests that the total load of virus in the host does not quite depend on the number of infections. That \beta is simply divided between the two strains in a host in proportion to their relative virulences also points to the same direction. But can't we imagine that the two infections are somewhat different in their location, and thus that a doubly infected host suffers more overall? To make it clearer: if the within-host dynamics is governed by pure resource competition, as the authors say, then either the two strains will not persist within the host (no coexistence) and two identical infections would not cause a great difference compared to a single infection (nothing would select for the second infection to increase in frequency within the host). The fact that this is not the case (coexistence + quantitative difference between the single and doubly infected hosts) implies on the contrary some form of niche differentiation (spatial, temporal or whatever) of pathogen populations within an individual host. This in turn would lead to the possibilities I mention above (Dmm not negligible, or \alpharr > \alphar). I would also think that imposing a strong limit on double infections (i.e. no host can be infected more than twice), even when, in the model simulations, most hosts can be in the twice-infected state (Figures 1 and 2), is strange if there is no niche differentiation of the consecutive infections (e.g., there are no more than two lungs in a body, to follow on my earlier example). Otherwise, multiple infections could readily occur and consecutive infections would add up into the total population as is modelled here. A common motivation to "cut" the vector of multiple infections is when multiple infections are rare (and thus beyond two infections, we can neglect the events). But this is obviously not the case in this model.
I do not ask the authors to provide an explicit equation for the dynamics of resource and competition within a host, but simply to clarify the type of within-host interactions at play and how the different assumptions would result from this interaction (especially since some sentences in the Discussion revolve around these issues).
-b- Why is the clearance rate \gamma is assumed to be the same for two strains competing in a host? If the two strains differ in virulence, and thus have different transmission rates from the host (\beta), I expect their respective loads differ within the host, so that the "rarer" variant (rare because of competition from the other variant) might also be more susceptible to disappear from the host?
-c- In Section S3, when introducing a virulence-transmission tradeoff, how exactly is the function \beta(x,c) combined with eq (2) in the main text? The base model assumes the two strains share a common pie (\beta) in proportion to x, but how do you do this in the more complex model where the two pies differ in size? Please clarify.
-d- On the formatting of equations and presentation of model: in many cases, parameters are represented by one letter without a subscript, which strongly suggests they are constant, and it is only much alter that we learn that, in fact, the parameter is not constant but is dependent of various things. This is confusing and should be changed so that we can immediately see which parameters are the same or may differ. Typically, in eq (1), it looks like \alpha and \gamma will be constant, as it is not subscripted. It is not clear until much later (e.g. eq (5-6)) that the two will vary. The same thing holds for \beta, actually.
-e- On the definition of the fitness and adaptive dynamics: the section presenting the fitness R and its subsequent use for evolutionary analysis (page 10) is extremely elusive. I could not find anywhere what R looks like exactly. It is mentioned that it is obtained from a local stability analysis, but I'd like to see more details, and perhaps an expression for R (which is usually feasible in those types of models, as a R0-like metric), that I could not find in the main document or in the Supp. Material. In the same vein, it is stated l. 94 that the equilibrium can be obtained analytically, but this is shown nowhere and never used: either show the result, or the statement can as well be dropped.
Also, the authors never model the joint evolution of the two traits but perform two one-trait ESS analyses and "superpose" them. Did they check the convergence stability in two-dimension? Is the co-ESS a stable node or a focus? Can there be evolutionary cycles?
-f- On line 159, the conditions for convergence stability and branching are exchanged: the first condition b<0 ("R is at a local maximum") is for evolutionary stability and the impossibility of being invaded, whereas the second a-b>0 is for convergence stability, contrary to what is written.
TYPOS and other side points
-- Line 195: " focusing on the prevalence of co-infections alone is not enough to predict how ESI will evolve." What do you mean exactly by prevalence of co-infections? Do you mean the ratio D/(D+I) or just D? It seems that the ration D/(D+I) is stricttly decreasing with virulence, and that the switch in the gradient of Immuno Suppression coincides with a 50:50 ratio (D/I=1), so that the latter has some utility to predict the change in ESI.
l. 104: the more virulENT strains... l. 97: the first sentence of this section is not very clear, please reformulate. l. 20 in Supp. Material: The Figure called should be S2, not S1 l. 38 in Supp. Material: same thing
Reviewed by anonymous reviewer 1, 07 Jul 2017
In this work, the authors apply an adaptive dynamics framework based on epidemiological models to find how the presence of immunosuppression affects virulence evolution in the context of multiple infections. As a result they find that by increasing the opportunity of co-infections, the presence of immunosuppression leads to ESS with higher virulence levels. This effect is modulated by factors such as the benefit from reduced clearance or host background mortality such that the optimal immunosuppression will depend on virulence in a non-simple way and also on the specific trade-offs between recovery rate and increased susceptibility of hosts to co-infections. This is a very interesting work and the approach taken, even if based on a specific set of epidemiological assumptions, adequate to produce results of relevance to the study of evolution of pathogenesis.
I only have some comments regarding parts of the manuscript where more details are needed or clarifications should be given.
1.Lines 15-16 partially repeat lines 12-14 in abstract.
2.The placement of equations 2 in the text is awkward. Those formulas are only mentioned much later in the text.
- Even if the base model is already described in Alizon 2008, and given the importance of the epidemiological assumption of the Drr individuals, the comparison between their resident dynamics to the mutant system should be made more explicit. For instance, it is stated that: "We treat the host classs Drr similarly to singly infected hosts, Ir, except for the fact that the doubly infected hosts cannot be infected any further." (lines 93-95) I guess this only refers to to the risk of contracting a further infection. What about mortality rate? From line 141 one would conclude that this is resolved by assuming a mean alpha of the infecting strains, which seems to differ from Lipsitch et al 2009 approach. Related to this, the authors have probably checked that the augmented models (equations 4 and equations 5 and 6) lead to the same equillibria as the one with just the resident allele (equations 1), if the mutant allele is absent. If so, this should be mentioned.
4.The equilibrium demographics under immunosuppression are only shown in the context of co-evolutionary stable immunosuppression (co-ESI) or co-evolutionary stable virulence. But how much those demographics differ from the ones obtained under the resident strain only?
- Why aren't the formulas for the dynamics of the susceptible individuals shown for the mutant systems? Given the focus that is given later on the demographics of the different individual types (particularly Fig 2) it would be better if those would be included (even with the cost of some repetition)
6.Regarding virulence evolution (Figure 1), why is that at immunosuppression approaching 100% there is a sharp increase in the ESV? What are the equilibrium frequencies of S,I and D in that situation? Does it still conform to the explanation that the increase in ESV is due to more opportunity for within-host competition as proposed by the authors?
For the immunosuppression evolution. When virulence is increased is just the virulence of the resident strain (and the mean virulence, as expected from page 9) while maintaining the virulence of possible mutants the same?
Line 211. Force of infection or virulence?
The way the whole paragraph that includes lines 210 to 219 is presented is somewhat confusing. I guess this stems from not being immediately clear to which part of the graph the authors are referring to in lines 211 to 213 (it must be the left part of the graphs in Figure 2).
Color scale in Figure 3 refers to ESI (a) and ESV (b) obtained, but this not clear in the Figure or Figure legend.










